One of the constant threads of my work from the start has been the curious gaps between the world our minds present to us as a whole, and the one nature builds for us to *work* as a whole (using a considerably more complete deck of cards, you might say)… Here’s a good note to a mathematician and physicist, John Baez on his Azimuth website, prompted by the interest he expressed in studying the math of real world problems in his comments regarding mathematical economics.

John, in your first comment at the top I noticed your intent to go back to teaching math differently next time, as you said:

“but now I’m more keen on real-world examples that illustrate the big problems facing our civilization..”

What could be challenging and intriguing is to try to model the elemental problem of “complex systems with thinking parts”, or “learning systems”.

The problem is how a network of independently “observing, interpreting & responding” agents would interact.   They are not follow rules, exactly, but following discoveries instead.

Learning systems share their own original learning and responses and developed a combined viewpoint of their dynamically changing environment.  Economic markets do that for example.

Their “thinking nodes” are key working parts in all kinds of natural system economies, responsible for swarm behaviors in nature generally, and not just in human economies. Whether their connections are direct through open markets or through specialized groups (like this blog, say), the interesting ways nature uses that structure to get distributed systems to work as rapidly reorganizing whole systems is a big part of the fascination. To avoid some of the indeterminacy of it all one starting point is to focus on the natural limitations of the actors or their environment, i.e. response time limits.

It would be interesting to model conditions in which smooth flowing learning and communication becomes turbulent, for example. That happens easily when the delay or difficulty in observation leads to interpretation uncertainty, and in turn leads to response errors like indecision or panic etc… See my idea? Maybe if people learn to play with idealized examples of this kind of thing it would give them a leg up for learning how to observe active learning system behaviors in real time they’d otherwise not think twice about.

There was a great example of an emerging systemic distortion developing in the collective “thinking system” of the financial markets in 2007, displayed in the S&P 500 index, that kind of points to the subject. This is just one of a wide variety of other kinds and scales of emergent learning system behaviors, growth itself being both the simplest, most common and most difficult to understand.

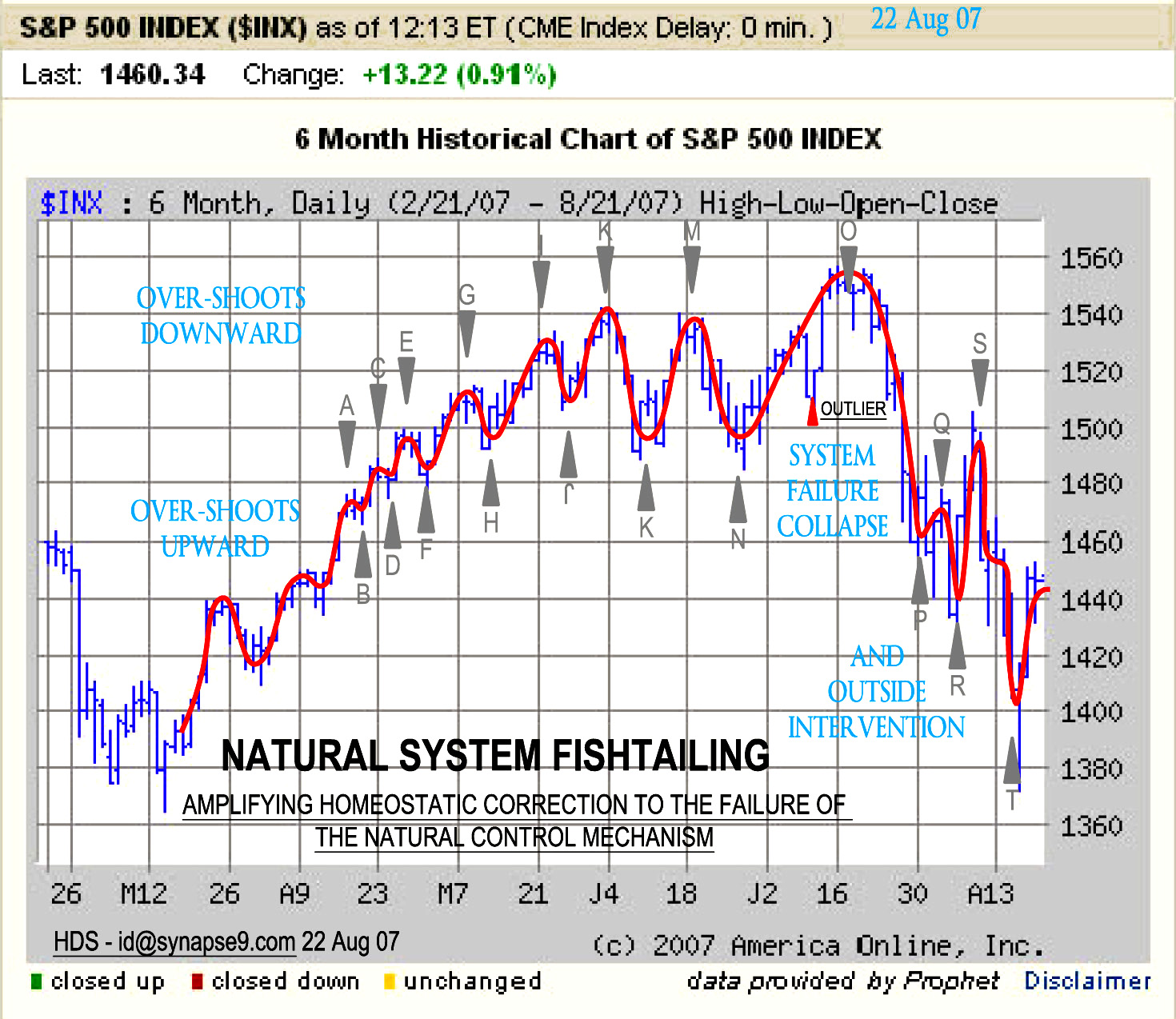
The screen shot shows the six month period from March to August, displaying increasing “fishtailing” leading to turbulence and the S&P whole market plunge, that triggered global intervention. I found lots of people who indeed agreed they’d never seen anything systemic like this, and that a functioning market should never produce them. I think what was driving it was that at that time people in the financial markets were racing all over looking for where to hide their money… they were panicking.

Another part of what’s interesting about it is how a highly exceptional systemic behavior did not stimulate the curiosity of the economists or traders I could find. All I could get was an opinion that it didn’t look right. That “failure of curiosity” hints at the deeper problem, of course.

That time the intervention by the market system regulators was able to save the day. That was not the case the next time “all hell broke loose”, though.

That people “in the know” didn’t take interest in the problem, of course, is another problem when relying on the “observing, interpreting & responding” of independent agents. They can look at something unfamiliar and “draw a blank”.

Anyway, that might help suggest the general lines of research into such emergent phenomena I’m trying to suggest. Various pieces of it could be experimented with mathematically, and give people an idea of what to be curious about, and “if you see something say something” if they happen to notice them taking place.

Phil

p.s.
I don’t know how to do the math, really, but you might. What I was suggesting in my comment yesterday on your interest in real world problems for Mathematical Economics is a form of modeling for economic systems somewhat like QM.

The difference from QM for studying the boundary conditions for large “observation, interpretation & response” networks (that economies are built around) would be in the modeling of the “mysterious hidden process” connecting the “before” and “after” states. For learning machines that process would have variable non-zero duration as well as uncertainty (in this case for altogether human rather than mathematical reasons).

That would let you explore some of the natural limitations of using such an operating system to run a planet. These kinds of structures proliferate in economies and other natural systems, especially those that exhibit rapid organizational development processes. So the work would be both innovative and possibly quite valuable.